Authorized National Elite Distributor
for the SMC Corporation of America
Authorized National Elite Distributor
for the SMC Corporation of America
We build the type of instruments we would love to use. This has made us successful for the last twenty years. Whether you’re ordering our mass flow or pressure instruments, you’ll receive a product that is a joy to use, backed by a company that is proud to help.
Gas and liquid flows (fluid flows) can be described as being in one of three states; turbulent, transitional, or laminar.
Turbulent flow
Turbulent flow is by nature chaotic. The fluid mixes irregularly during turbulent flow. Constant changes in the flow’s behavior (wakes, vortexes, eddies) make flow rates difficult, if not impossible, to accurately measure. Turbulent flow usually occurs at high flow rates and/or in larger diameter pipes. Turbulent flow is usually desirable when solids must remain suspended in the fluid to prevent settling or blockages.
Transitional flow
Transitional flow exhibits characteristics of both laminar and turbulent flow. The edges of the fluid flow in a laminar state, while the center of the flow remains turbulent. Like turbulent flows, transitional flows are difficult, if not impossible, to accurately measure.
Laminar flow
Laminar flow layers
Laminar or Smooth flow tends to occur at lower flow rates through smaller pipes. In essence, the fluid particles flow in cylinders. The outermost cylinder, touching the pipe wall, does not move due to viscosity. The next cylinder flows against the unmoving fluid cylinder, which exhibits less frictional “pull” than the pipe wall. This cylinder will move the slowest. This continues, with the centermost cylinder having the greatest velocity.
How do we know if a flow is turbulent, transitional or laminar? In the late 1800’s, Osbourne Reynolds discovered that the type of a fluid flow is related to the fluid’s density, mean velocity, diameter and viscosity. This dimensionless (no units) number helps predict changes in flow type. In simple terms, the Reynolds Number can be written as:
density x mean velocity x diameter / viscosity
It is generally accepted that flow is laminar if the Reynolds Number is less than 2000. Transitional flows have a Reynolds Number between 2000 and 4000. Flows are considered turbulent when the Reynolds Number is greater than 4000. Using the Reynolds equation, we can see that reducing the density, mean velocity and/or diameter of a turbulent fluid flow (unchanging viscosity) will make it “more” laminar. This could also be accomplished by increasing the fluid viscosity (keeping density, mean velocity and diameter the same). The inverse is true to make a flow more turbulent.
Pressure drop describes the loss of pressure as a fluid travels through a pipe or channel. If you blew into a mile long pipe, it’s unlikely that anything would come out the other end. This is due to pressure drop. As the fluid flows through the pipe, friction with the pipe walls and between the fluid particles causes a loss of pressure. Pressure drop is approximately proportional to the distance the fluid travels.
Mass is a measure of the amount of matter that makes up an object. The mass of an object is considered constant. Volume refers to the amount of space an object takes up. The volume of an object can change depending on pressure, temperature and other factors. In terms of flow, at room temperature and low pressures the volumetric and mass flow rate will be nearly identical, however, these rates can vary drastically with changes in temperature and/or pressure because the temperature and pressure of the gas both affect the volume. For example, assume a volumetric flow reading was used to fill balloons with 250 mL of helium, but the incoming line ran near a furnace that cycled on and off, intermittently heating the incoming helium. Because the volumetric meter simply measures the volume of gas flow, all of the balloons would initially be the same size. However, if all the balloons are placed in a room and allowed to come to an equilibrium temperature, they would generally all come out to be different sizes. If, on the other hand, a mass flow reading were used to fill the balloons with 250 standard mL of helium, the resulting balloons would initially be different sizes, but when allowed to come to an equilibrium temperature, they would all turn out to be the same size.
Alicat Whisper-series flow meters and controllers are intended for use in low-pressure applications. This is because an accurate measurement of the volumetric flow rate by means of differential pressure requires the flow at the differential pressure sensor to be in a laminar state. The state of the flow is quantified by what is known as the Reynolds Number. If the Reynolds Number gets above a certain quantifiable point the flow will become non-laminar. Most Alicat volumetric flow devices are sized to make valid full-scale measurements with line pressures up to 10 – 15PSIG when using air.
As a general rule, if your line pressures will be above 15PSIG, an Alicat mass flow device will be more appropriate due to the additional sensors required to compensate for the increased densities.
Choosing the right mass flow or volumetric flow device can mean the difference between your process working or working to it’s full potential.
Learning about mass and volumetric flow devices will not cost you anything. However, selecting a mass or volumetric flow device without learning about them could lead to a significant cost in both time and money.
Flow instrumentation does not look very different on the surface, but most devices operate quite differently. It is important to know what you require from a flow device before you ever decide to buy a flow device. There are a number of options and features that differ from manufacturer to manufacturer. At the end of the day, a flow device is a measurement tool. Prior to shopping for a device you should know the following about your own process measurement requirements to ensure that you are shopping for the right device:
All of this information will be important in helping you choose the right flow device for your process. Different device manufacturers have different specifications for their devices. Some will operate under higher pressure or temperature conditions than others, some respond faster to changes in flow than others and so on.
Flow devices generally follow one of several principles. Each of these methods has distinct advantages and disadvantages. While one type of device may flow a gas at high temperature or high pressure it may not do so very accurately, or vice versa. If high temperature, high pressure and accuracy are all critical elements in your process than it is important to choose a device that adequately meets all three requirements. However, if one requirement is more important than the others a different device may be selected.
It is also very important to decide early on what features and characteristics will be of most use to you for your particular project. A flow device that comes with a standard display may be of great value if the process must constantly be monitored, but would be of no value if it did not flow the type of gas that your process requires. Some manufacturers include more standard features than others. If you decide on a certain brand of device, as it meets the criteria of your process, also make sure that you will not have to purchase additional ‘options’ that will make the device compatible with your process. Many manufacturers charge for multiple outputs, digital communication, displays, valve types, seals, fittings, etc. It is important to know what you require and choose a device in which most of these options are standard to keep costs to a minimum.
Understanding specs is very important when shopping for a flow device. You will be presented with specification sheets that indicate the physical and operational characteristics of a flow instrument. These spec sheets are designed to describe the operating characteristics of the device. It is important to know what those specifications mean and how they apply to your process or measurement goal. Select the “Understanding Specifications” tab below to learn more about specifications and what they mean.
Alicat mass flow instruments are founded on the principles of this measurement method, and take full advantage of the many intricacies required to measure this accurately.
One methodology for an Internally Compensated Laminar (ICL) unit is based on the physics of the Poiseuille Equation. First an internal restriction is created. This restriction is known as a Laminar Flow Element (LFE). The LFE forces the gas molecules to move in parallel paths along the length of the passage, nearly eliminating flow turbulence (see the “Flow Principles” tab above). The differential pressure drop is measured within the laminar region. The Poiseuille Equation quantifies the relationship between pressure drop and flow as:
Q = (P1 – P2)p (r^4) / 8?L
Where:
Q = Volumetric Flow Rate
P1 = Static pressure at the inlet
P2 = Static pressure at the outlet
r = Hydraulic Radius of the restriction
? = (eta) absolute viscosity of the fluid
L = Length of the restriction
Since p, r and L are constant, the equation can be rewritten as:
Q = K(? P/?)
In this equation, K is a constant factor determined by the geometry of the restriction. It shows the linear relationship between volumetric flow rate (Q), differential pressure (?P), and absolute viscosity (?) in a simpler form.
Changes in gas temperature affect the absolute viscosity of the gas. This requires a temperature measurement to determine the value of ?. For most DP devices this is done by manually referencing charts that indicate the viscosity properties of the gas at given temperatures. In an ICL device this reference is performed internally through the use of a discrete temperature sensor and a microprocessor.
At this point only the volumetric flow rate has been determined. For an ICL device to address the range limitations of thermal devices, additional measurements must be taken to determine the actual mass flow rate of the gas. The relationship between volume flow and mass flow is:
Mass = Volume * Density Correction Factor
Ideal gas laws show us that the density of a gas is affected by its temperature and absolute pressure. Using ideal gas laws, the effect of temperature on density is:
At constant pressure, ?a / ?s = Ts / Ta
Where:
?a = Density @ Flow Condition
Ta = Absolute Temperature @ Flow Condition in Kelvin
?s = Density @ Standard Condition
Ts = Absolute Temperature @ Standard Condition in Kelvin
°K = °C +273.15 (to find Kelvin)
And the effect of absolute pressure on density is:
At constant temperature, ?a / ?s = Pa / Ps
Where:
?a = Density @ Flow Condition
Pa = Flow Absolute Pressure
?s = Density @ Standard Condition
Ps = Absolute Pressure @ Standard Condition
Therefore, in order to determine the mass flow rate (M), two correction factors must be applied to volumetric flow rate (Q): temperature effect on density, and absolute pressure effect on density. This can be written as:
M = Q(Ts / Ta)( Pa / Ps)
In an ICL flowmeter a discrete absolute pressure sensor is also placed in the laminar region of the flow stream. This information is sent to the microprocessor and is combined with the data from the discrete absolute temperature sensor for the appropriate calculations to determine mass flow.
Performing these calculations requires reference to some standard temperature and pressure (STP) as indicated by variables Ts and Ps. STP is usually defined at sea level conditions, but no single standard exists for this convention. Examples of common reference conditions include:
0 °C and 14.696 PSIA
25 °C and 14.696 PSIA
0 °C and 760 torr (mmHG)
It is relevant to note, while the correct units for mass are expressed in grams, kilograms, etc., it has become standard that the mass flow rate is specified in SLPM (standard liters per minute), SCCM (standard cubic centimeters per minute) or SCFH (standard cubic feet per hour). By knowing the STP calibration of the device and the density of a particular gas at that STP, it is possible to determine the flow rate in grams per minute, kilograms per hour, etc. For example:
Given:
Gas = Helium
M = 250 SCCM
STP = 25 °C and 14.696 PSIA
Gas Density = 0.166 Grams per Liter
True Mass Flow = M * Gas Density at STP
True Mass Flow = (250 SCCM)(1 liter per 1000 CC)(0.1636 grams per liter)
True Mass Flow = 0.0409 Grams per Minute of Helium
Thermal mass flow controllers were originally developed in the 1960s and 1970s for the semiconductor industry for gas vapor deposition in semiconductor fabrication.
As time went on the thermal mass flow controller found its way into other processes such as pharmaceutical drug discovery, leak testing and aerospace. Mass flow controller and mass flow meters are now used in any application that requires the precise control and measurement of process gasses.
Thermal technology is based on the principles of thermal convection and anemometry, the measurement of wind speed. Dating to the 1600s, anemometer technology was significantly improved in the early 1900s and is still widely used today.
As the demand had risen for mass flow meters and mass flow controllers, so did the demand for expanded functionality in mass flow meters and mass flow controllers. Consumers required increased accuracy, increased speed of response, more features and easier operation. The expanding market for mass flow meters and mass flow controllers created new demands for existing thermal technology. Operating characteristics inherent to thermal technology such as long warm up times and slow response speed began to create an opportunity for new technologies to enter the mass flow market. Inventors began finding new opportunity in the mass flow market, and new technologies like laminar differential pressure measurement slowly began to be introduced. Along with these new technologies, thermal mass flow meters and mass flow controllers have continued to evolve.
| Alicat Mass Flow Devices | Typical Thermal Mass Flow Devices | |
|---|---|---|
| Sensor | Solid-State Silicon Based Differential Pressure | RTD or Thermocouple |
| Response Speed | 10 milliseconds (no software corrections required) for flow meters and 50 milliseconds for flow controllers | 0.5-3.0 seconds (no software), 500 milliseconds (software corrections predict flow) |
| Display | Standard, Integrated or Remote | Optional if available, External Mount |
| Totalizer | Optional, Integrated | Optional if available, External Mount |
| Process Data | Integrated Display shows Mass Flow Rate, Volumetric Flow Rate, Line Temperature and Line Absolute Pressure | Mass flow rate |
| Output Options | Standard integrated display, analog (either 0-5 Vdc, 0-10 Vdc, 1-5 Vdc, or 4-20mA), and Standard RS-232 (no special software required). Optional 2nd analog output can be ordered to output either mass flow, volumetric flow, temperature, or absolute pressure. |
Standard analog, optional display if available, optional digital output if available. |
| Digital Output | Standard output includes mass flow rate volumetric flow rate, line temperature, line absolute pressure, selected gas, AND total if ordered with totalizer option. | Digital output of mass flow if available. |
| Power | 7-30 Vdc, 35mA for flow meters. 12-30Vdc 250mA for small valve controllers and 24-30Vdc and 750mA for large valve controllers. Standard AC/DC adapter jack AND cable connector pins. Can run off anything from an integrated rechargeable battery to 12 or 24 volt systems from supplies or an inexpensive wall plug adapter. | Special supply with + and – regulated 15 Vdc |
| Fittings | Standard NPT or miniature pneumatic fittings. Inexpensive, adaptable to common components. Swage-lok® style fittings are also available upon request. | Specialized compression, VCR, etc. |
| Multi-gas Versatility | Standard suite of 98 gas calibrations, selected from integrated display. | Single gas, conversion charts |
| Inherent Linearity | Yes | No |
| Documentation | Integrated display shows model number, serial number, date of manufacture, calibration technician, and software revision number. Model/Serial number label also standard. | On paper included with unit, sticker |
| Flow Ranges | Ranges available from 0-0.5 standard cubic centimeters/min to 0-5000 standard liters/min at full scale. | Ranges available from 10 standard cubic centimeters/min to 50 standard liters/min full scale. |
Alicat Scientific manufactures mass flow meters with laminar differential pressure measurement technology, which is one of many types of flow measurement techniques. This article provides a brief overview of the primary methods for measuring gas flow used today.
Laminar flow meters use the pressure drop created within a laminar flow element to measure the mass flow rate of a fluid. A laminar flow element converts turbulent flow into laminar flow by separating it into an array of thin, parallel channels. The decrease in pressure, or pressure drop, across the channel is measured using a differential pressure sensor. Because the flow is not turbulent, but laminar, the Poiseuille Equation (see the “Multiple Parameter Laminar Flow” tab above) can then be used to relate the pressure drop to the volumetric flow rate. The volumetric flow rate can also be converted to a mass flow rate using density correction at a given temperature and pressure.
Alicat Scientific offers a range of mass flow meters and mass flow controllers for gas flow, as well as liquid flow meters and controllers, that operate via laminar differential pressure measurement.
As the name implies, thermal flow meters use heat to measure the flow rate of a fluid. Thermal flow meters traditionally work in one of two ways. The first type measures the current required to maintain a fixed temperature across a heated element. As the fluid flows, particles contact the element and dissipate or carry away heat. As the flow rate increases, more current is required to keep the element at a fixed temperature. The current requirement is proportional to the mass flow rate. The second thermal method involves measuring the temperature at two points on an element or ‘hot wire’. As the fluid flows over the element it dissipates heat. The upstream side of the element will be hotter than the downstream side. The change in temperature is related to the fluid’s mass flow.
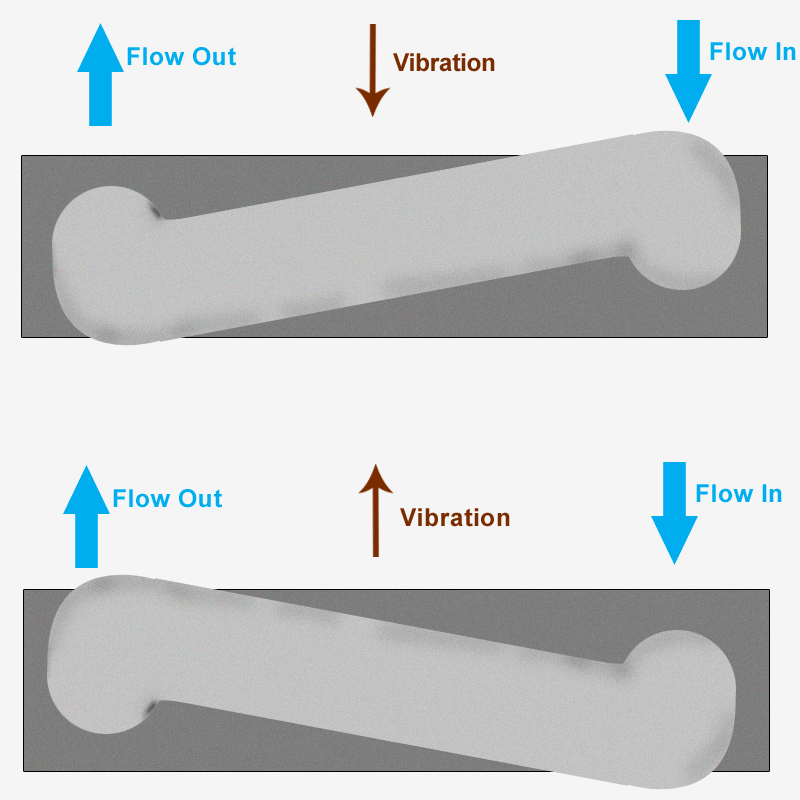The Coriolis flow meter uses the Coriolis Effect to measure the mass flow of a fluid. The fluid travels through single or dual curved tubes. A vibration is applied to the tube(s). The Coriolis force acts on the fluid particles perpendicular to the vibration and the direction of the flow. While the tube is vibrating upward, the fluid flow in forces down on the tube. As the fluid flows out of the tube, it forces upward. This creates torque, twisting the tube. The inverse process occurs when the tube is vibrating downward. These opposing forces cause the tube to twist, the amplitude of which is directly related to mass flow of the fluid through the tube.
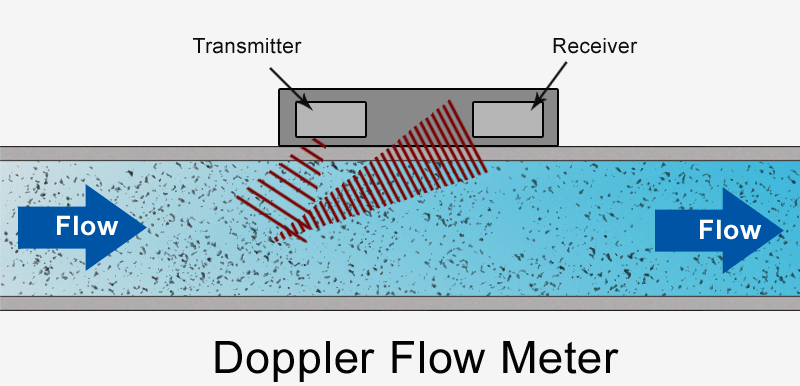
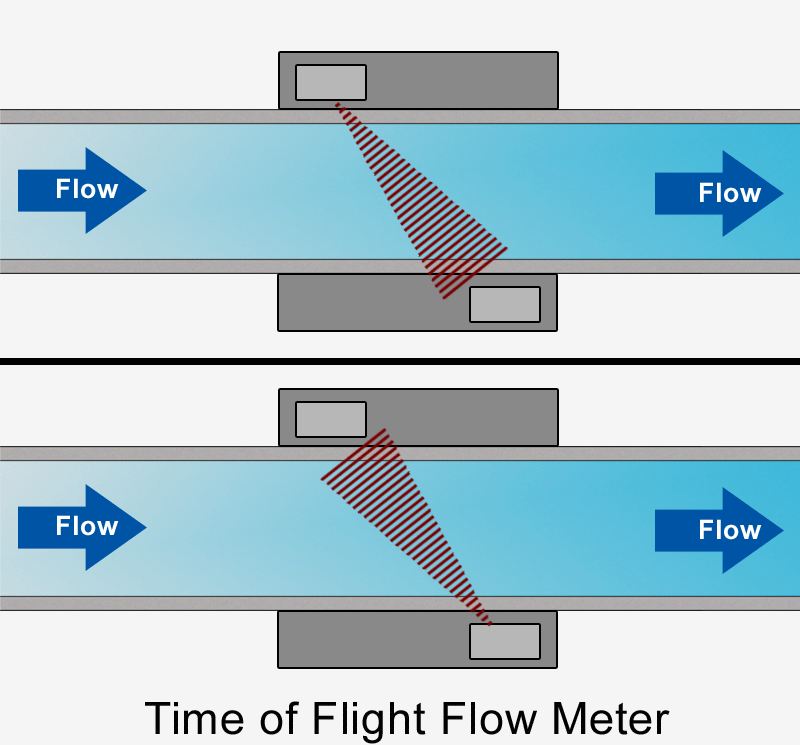Ultrasonic flow meters use sound waves to measure the flow rate of a fluid. Doppler flow meters transmit ultrasonic sound waves into the fluid. These waves are reflected off particles and bubbles in the fluid. The frequency change between the transmitted wave and the received wave can be used to measure the velocity of the fluid flow. Time of Flight flow meters use the frequency change between transmitted and received sound waves to calculate the velocity of a flow.
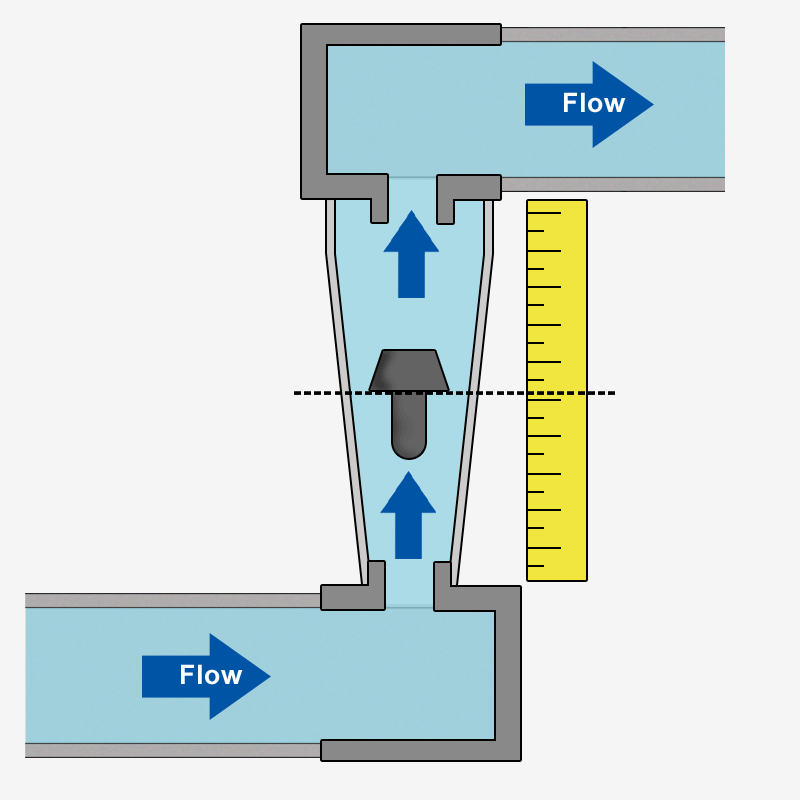Variable area flow meters, or rotameters, use a tube and float to measure flow. As the fluid flows through the tube, the float rises. Equilibrium will be reached when pressure and the buoyancy of the float counterbalance gravity. The float’s height in the tube is then used to reference a flow rate on a calibrated measurement reference.
When you are looking at a spec sheet for mass flow meters and mass flow controllers the amount of information presented can be a little overwhelming. Especially, if you are not familiar with what all of the terminology means or if you are not sure which of the specs will have the most impact on what you are trying to accomplish.
Listed below are some of the more important mass flow meter and mass flow controller specs and what they mean in plain english.
Accuracy is a measurement of how accurately an instrument performs at different flow ranges.
Accuracy is generally measured in one of two ways: percentage of full scale flow or percentage of reading.
Error as a percentage of full scale is established by multiplying the error percentage by the full scale flow. The less you flow through the device the less accurate the reading will be. For that reason, you don’t want to get a larger device than you need. Devices with error expressed as a percentage of full scale are most accurate when flowing at full scale.
Error expressed as a percentage of reading expresses error as a percentage of what the device is actually flowing. Simply, if a instrument’s accuracy is rated to +/-1% of reading an instrument will be accurate to +/-1% of whatever the instrument is flowing. At 100SLPM the instrument will be accurate to within +/-1SLPM, and at 10SLPM of flow the unit will be accurate to within +/-.1SLPM. This way of measuring accuracy usually accompanies a full-scale statement of accuracy, for example +/-(0.8% of reading + 0.2% of full scale). Total accuracy requires adding the two terms at the flow rate in question.
Accuracy, regardless of measurement method, is generally dependant on operating conditions. Operating conditions are usually defined as the pressure and temperature of the gas flowing through the instrument. Manufacturers will rate their instrument’s error based on some predefined set of operating conditions, usually standard pressure and temperature. So, if your gas temperature and/or gas pressure do not meet those conditions specified by the manufacturer the accuracy of your unit could be off by quite a bit. Some units, like Alicat’s, are internally compensated which means that sensors inside the device measure temperature and pressure conditions and make real time corrections for variations in gas conditions. Real time corrections for variations in gas conditions take a lot of worry about maintaining consistent process conditions.
Repeatability measures an instrument’s ability to repeat flow functions accurately.
A unit’s repeatability is generally measured by monitoring a flow instrument’s reading at a given flow rate, turning off the flow allowing instrument to return to zero for a given period of time, and then resuming the same flow. The instrument’s repeatability is determined by examining the difference between the original flow reading and the flow reading after the flow has been turned off and resumed.
Simply, repeatability measures how repeatable an instrument’s reading will be at the same flow rate.
Zero shift or offset shift is defined as how far from zero an instrument will move when pressure and/or temperature are changed. Offset error does not affect the slope of the calibration curve, any offset error will be the same throughout the flow range. Offset error is measured in %FS (or %reading)/degree change in temp (or psi change in pressure) Simply, for every change in degree temp or change in psi the calibration is offset by the percentage of error.
Span shift or span error is defined as a shift in the slope of the calibration curve with zero not changing. The calibration curve of the device will be affected differently at different flow ranges. Span error is measured in %FS (or %reading)/degree change in temp (or psi change in pressure) Simply, for every change in degree temp or change in psi the calibration is offset by the percentage of error
Zero shift or span shift can also be referred to as ‘Temperature coefficients’ or ‘Pressure Coefficients’ and will be measured the same way. Be sure to pay attention to the units of measure as some manufacturers will measure span or offset error percentages by measuring in degrees F or single psi instead of degrees C or atm’s of pressure.
Turndown ratio is a measure of the useable range of an instrument, expressed as the ratio of maximum flow to minimum flow. Simply put, it is the minimum amount of fluid that can be measured by the device. Turndown ratio indicates how much of the instrument range can produce accurate readings, which is very important when you want to measure or control a very wide flow range without having to change instruments.
Warm up time measures the amount of time it takes for an instrument to become stable for use. Thermal units tend to have the longest warm up times. Some units can take up to 30 minutes to become stable to within 2%FS. This is an important specification if you turn your unit off at the end of the day.
Pressure drop describes the loss of pressure as a fluid travels through a pipe or channel and any instruments along the way. If you blew into a mile long pipe, it’s unlikely that anything would come out the other end. As the air flows through the pipe, friction with the pipe walls and between the gas particles causes a loss of pressure. Pressure drop is approximately proportional to the distance the gas travels. Every component that comes in contact with the gas–every instrument, fitting, bend, pipe wall, etc.–induces some pressure drop.
Since pressure drop is a flow killer, gas processes that have little available differential pressure are best optimized by making sure that every component in the system generates as little pressure drop as possible. Alicat’s Whisper Series of low pressure drop gas flow meters and controllers can help.
Dead band is defined as an area of a signal range or band where no action occurs. Put simply, the band where the system is dead.
Dead band as it relates to a pressure switch is the band in between which the switch trips (the setpoint) and where the switch resets.
Gas Viscosities, Densities, and Compressibilities at 25°C PDF (43 kB)
Gas Viscosities, Densities, and Compressibilities at 0°C PDF (43 kB)
Get Adobe Reader
| Molecular Weight | Density | ||
|---|---|---|---|
| Gas | Grams/Mole | Grams/Liter at 0°C, 29.92? Hg |
Grams/Liter at 25°C, 29.92? Hg |
| Butane | 58.124 | 2.5932 | 2.3758 |
| Propane | 44.097 | 1.9674 | 1.8024 |
| H2 | 2.016 | 0.0899 | 0.0824 |
| Ethane | 30.070 | 1.3416 | 1.2291 |
| Acetylene | 26.038 | 1.1617 | 1.0643 |
| Methane | 16.043 | 0.7158 | 0.6557 |
| Nitrous Oxide | 44.013 | 1.9637 | 1.7990 |
| CO2 | 44.011 | 1.9636 | 1.7989 |
| CO | 28.010 | 1.2497 | 1.1449 |
| N2 | 28.013 | 1.2498 | 1.1450 |
| Air | 28.964 | 1.2922 | 1.1839 |
| He | 4.003 | 0.1786 | 0.1636 |
| O2 | 31.999 | 1.4276 | 1.3079 |
| Ar | 39.948 | 1.7823 | 1.6328 |
| Krypton | 83.800 | 3.7388 | 3.4253 |
| Neon | 20.183 | 0.9005 | 0.8250 |
A
The total of the indicated gage pressure plus the atmospheric pressure. Abbreviated “psia” for pounds per square inch absolute.
Quantity defining the limit that errors will not exceed. When applied to flow meters, accuracy is specified in either % of full scale or % of rate.
The pressure exerted upon the earth’s surface by the air because of the gravitational attraction of the earth. Standard atmosphere pressure at sea level is 14.7 pounds per square inch (psi). Measured with a barometer.
B
An instrument for measuring atmospheric pressure.
C
The Coriolis flow meter uses the Coriolis Effect to measure the mass flow of a fluid. The fluid travels through single or dual curved tubes. A vibration is applied to the tube(s). The Coriolis force acts on the fluid particles perpendicular to the vibration and the direction of the flow. While the tube is vibrating upward, the fluid flow in forces down on the tube. As the fluid flows out of the tube, it forces upward. This creates torque, twisting the tube. The inverse process occurs when the tube is vibrating downward. The amount of twist in the tube is directly related to mass flow of the fluid through the tube.
D
The difference between two pressures. Differential Pressure Flow Meters use differential pressure to compute volumetric flow rates.
Differential Pressure or Laminar flow meters use the pressure drop created within a laminar flow element to measure the mass flow rate of a fluid. A laminar flow element takes turbulent flow and separates it into thin channels. By reducing the diameter of the flow channel and affecting velocity, the flow becomes laminar through the channels. The decrease in pressure, or pressure drop, across the channel is measured using a differential pressure sensor. The Poiseuille Equation can then be used to relate the pressure drop to the volumetric flow rate. The volumetric rate can also be converted to a mass flow rate using density correction at a given temperature and pressure.
E
None Found
F
None Found
G
None Found
H
None Found
I
None Found
J
None Found
K
None Found
L
see Differential Pressure Flow Meter
M
Also called normal flow or standard flow. The mass flow rate can be thought of as what the volume flow rte would be if the gas flowing through the line were at standard conditions. Actual line pressure and temperature affect the density of the gas, which contracts (above atmospheric pressure and / or low temperature) or expands (under vacuum and / or high temperature), and thus affects the measured volume flow rate. This means that the exact same number of molecules of gas flow can be measured as radically different volume flows when the temperature or pressure is fluctuating. Some mass flow meters have an absolute pressure sensor, temperature sensor or other technique to determine and compensate for variable gas density on the fly. This means that a change in mass flow reading is known to mean an actual change in the number of gas molecules as opposed to a simple change in the gas density. In addition, when the volumetric flow rate is corrected to standard conditions, it is a simple matter to multiply the mass flow rate by the density of the gas at standard conditions (commonly published) to determine actual mass flow rate (e.g. grams / minute)
In reference to flow products or pressure products, media usually refers to the process gas or liquid to be used with the device.
Commonly used acronym for Mass Flow Controller.
N
All Alicat measurement and control instruments are furnished, at no extra cost, with a certificate of calibration. This certificate indicates the type of device, information about the customer who purchased the instrument and specific test data indicating the instrument’s performance in comparison to a known NIST traceable standard.
O
None Found
P
None Found
Q
None Found
R
Is the closeness of agreement between consecutive measurements of the same flow within a particular time frame. This can be specified as % of full scale or % of rate.
Smallest incremental change in a parameter that can be indicated on the display (Note: this is not the same as accuracy).
S
None Found
T
As the name implies, thermal flow meters use heat to measure the flow rate of a fluid. Thermal flow meters traditionally work in one of two ways. The first type measures the current required to maintain a fixed temperature across a heated element. As the fluid flows, particles contact the element and dissipate or carry away heat. As the flow rate increases, more current is required to keep the element at a fixed temperature. The current requirement is proportional to the mass flow rate. The second thermal method involves measuring the temperature at two points on an element or “hot wire”. As the fluid flows over the element it dissipates heat. The upstream side of the element will be hotter than the downstream side. The change in temperature is related to the fluid’s mass flow.
Turndown ratio is a measure of the useable range of an instrument, expressed as the ratio of maximum flow to minimum flow.
U
None Found
V
Indicates the actual volume of a gas. Since gases are compressible, the actual mass of the gas will be constant with temperature & pressure changes, but the volume will vary.
W
None Found
X
None Found
Y
None Found
Z
None Found